|
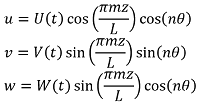 | (2) |
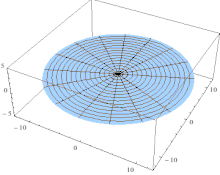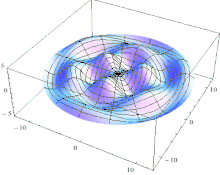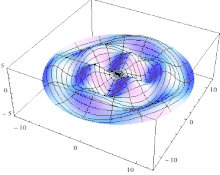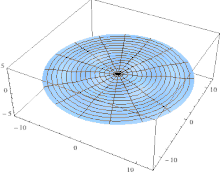
where the integers n and m determine the circumferential and the axial vibration modes, respectively. As a representative example, in Fig. 5 we show the first three lowest frequency modes.
For ![]() 1, the deviation of vibrational frequencies from those of a circular cylinder is of first order in
1, the deviation of vibrational frequencies from those of a circular cylinder is of first order in ![]() [67]. This allows us to write the equations of motion for the amplitudes U, V, W as
[67]. This allows us to write the equations of motion for the amplitudes U, V, W as
| (3) |
where x = (U, V, W), J0 is the Jacobian matrix corresponding to the circular cylinder and J1 is its first order correction due to squashing. When the solid plate is vibrated with the frequency ω, its vertical displacement is given by Acos(ωt), where A is the vibrational amplitude. The gravity acceleration in the co-moving frame of reference is g(t) = g(1 + αcos(ωt)), where α = ![]() is the dimensionless scaled amplitude. Since viscosity is neglected, we anticipate the onset of the subharmonic vibrations at small amplitude α ≪ 1. In this regime, we obtain from Eq. for the time-dependent squashing
is the dimensionless scaled amplitude. Since viscosity is neglected, we anticipate the onset of the subharmonic vibrations at small amplitude α ≪ 1. In this regime, we obtain from Eq. for the time-dependent squashing 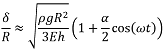 . In this limit, Eq. reduces to the three-dimensional Mathieu function
. In this limit, Eq. reduces to the three-dimensional Mathieu function
 | (4) |
where  is the scaled vibration amplitude.
is the scaled vibration amplitude.
We emphasise that the linear model Eq. can only be used for qualitative estimations of generally nonlinear subharmonic response. Discrepancies with experimental results obtained for real earthworms can be due to viscoelastic damping, nonlinear deformation and twisting of the worm body, as well as due to the assumption of a thin wall elastic cylinder filled with Newtonian fluid used in the model.
The properties of solutions of Eq. are well-known [68]. Parametrically excited instability sets in when 2πf coincides with one of the combination frequencies ![]() , where
, where ![]() are the eigenvalues of
are the eigenvalues of 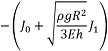 . Because ∼0.04 for E = 1 MPa, we can neglect the term
. Because ∼0.04 for E = 1 MPa, we can neglect the term ![]() J1 in Eq. as compared with J0, which implies that the natural frequencies ω0i of the gravity squashed worm can be approximated by those of a circular elastic cylinder.
J1 in Eq. as compared with J0, which implies that the natural frequencies ω0i of the gravity squashed worm can be approximated by those of a circular elastic cylinder.
Amongst the three frequencies (ω0) one is typically two orders of magnitude lower than the other two. This lowest frequency ω0 corresponds to the mode with predominantly radial displacement and is given by [64]
 | (5) |
where λm = ![]() and In is the modified Bessel function of the first kind of order n.
and In is the modified Bessel function of the first kind of order n.
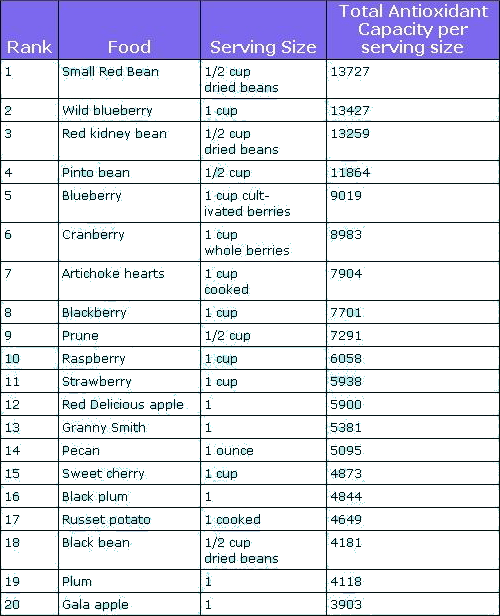
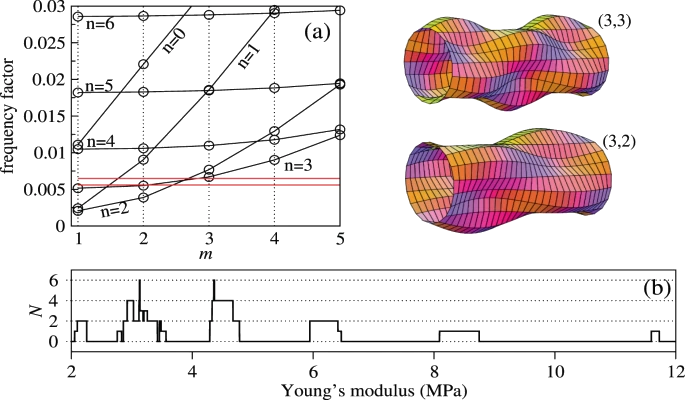
Considered as a whole, the combination ω0R![]() in Eq. depends on the geometry of the cylinder, the Poisson ratio ν and the ratio of the densities
in Eq. depends on the geometry of the cylinder, the Poisson ratio ν and the ratio of the densities ![]() , but is independent of the Young’s modulus E. In Fig. 6, we plot the frequency factor as a function of the axial mode number m for different values of n for a cylinder of length L = 10 cm, shell thickness h = 50 μm, radius R = 5 mm, filled with a fluid with density ρl = ρ = 1100 kg/m3. The first three lowest frequency modes with n = 1, m = 1, n = 2, m = 2 and n = 2, m = 1 are shown in the right panel of Fig. 5 for the sake of illustration.
, but is independent of the Young’s modulus E. In Fig. 6, we plot the frequency factor as a function of the axial mode number m for different values of n for a cylinder of length L = 10 cm, shell thickness h = 50 μm, radius R = 5 mm, filled with a fluid with density ρl = ρ = 1100 kg/m3. The first three lowest frequency modes with n = 1, m = 1, n = 2, m = 2 and n = 2, m = 1 are shown in the right panel of Fig. 5 for the sake of illustration.
Discussion
The presented theoretical model can, in general, be used to find the mode profiles corresponding to the resonance frequencies found in the experiment in Fig. 4 by using experimental data for the Young’s modulus E of earthworms as a key input parameter. However, plausible values of E for different worm species are a subject of active debate due to a large range of the reported values and poor understanding of the impact of the cuticle on mechanical properties of worms [53, 60].
To circumvent the lack of experimental data, we reanalyse our experimental results in Fig. 4 in light of the predictions of the developed model Eqs. In particular, we establish which of the theoretically possible vibrational modes could be excited at a given value of E. Here, we vary the value of E in a range bounded by two critical values – the effective bulk Young’s modulus of the worm from [53] and the locally measured stiffness of the cuticle [60, 61]. Whereas the exact values of E are yet to be confirmed experimentally, it has already been established that E would be a function of the thickness of the cuticle60 and that it would approach 200 … 400 MPa [61] in a limiting case of the mechanical properties of the worm defined solely by the cuticle.
Naturally, the thickness and stiffness of the cuticle vary for different species of worms and they are also likely to vary from one animal to another within the same species group. Indeed, in our experiments we established that Eisenia fetida earthworms appear to be slightly stiffer when palpated as compared with the other species tested in this work. However, this difference alone cannot result in an order of magnitude discrepancy in the values of E.
Anna Henschel. The Good, the Weird and the Hilarious Scientific Papers

A researcher of the study courageously jumping with an empty backpack. Picture taken from Yeh et al, 2018. Reproduced from the Open Access article distributed in accordance with the Creative Commons Attribution Non Commercial (CC BY-NC 4.0) license, which permits others to distribute, remix, adapt, build upon this work non-commercially, and license their derivative works on different terms, provided the original work is properly cited and the use is non-commercial. See: http://creativecommons.org/licenses/by-nc/4.0/
Prospective participants were approached and screened by study investigators on commercial or private aircraft. […] Typically, passengers seated close to the study investigator (typically not known acquaintances) would be approached mid-flight, between the time of initial seating and time of exiting the aircraft. […] All participants were asked whether they would be willing to be randomized to jump from the aircraft at its current altitude and velocity.
Parachutes and presumptions
References
Thébaud, O., Link, J.S., Kohler, B., Kraan, M., López, R., Poos, J.J., … & Handling editor: Howard Browman. Managing marine socio-ecological systems: picturing the future. ICES Journal of Marine Science, 74, 1965 — 1980.
Yeh, R.W., Valsdottir, L.R., Yeh, M.W., Shen, C., Kramer, D.B., Strom, J.B., … & Nallamothu, B.K. Parachute use to prevent death and major trauma when jumping from aircraft: randomized controlled trial. BMJ, 363, k5094.
Anna Henschel. Science has a Mean Girls Problem
The subtle bullying in the 2004 instant classic Mean Girls took place in an analogue world. Since then, we have left high school and entered the digital realms — where the spirit of the Plastics lives on.
This most recent Twitter discussion was sparked when the British Education Secretary Damian Hinds remarked that a degree in Psychology would be of low value, as some statistics suggested that graduates would not be able to repay their student loans within the first 5 years of completing their education. This echoed the tune of a familiar song: there seems to be an implicit pecking order of what is considered the most important and legit kind of science.
How did we get here?
I know you don’t think this is proper science, but believe me, this is what is considered the gold standard in my field.

Whenever I venture outside of my small area of expertise, I encounter people who, with great passion, share their thoughts and their enthusiasm for a topic they are investigating. Sometimes the problem is so intricate that it can be challenging to communicate to a non-academic audience, why this thing is so exciting. However, this should not be the case for fellow academics. As humans, we have a unique ability to empathize with others — regardless of their scientific specialization.
I can empathize with any researcher passionate about their subject, I can feel the pain of anyone who has worked for a long time on a difficult question and celebrate with colleagues outside of my lab who experience the joy that comes from discovering something new. For me, and I would argue any scientist, this should not be a big leap to make.
Just because a certain field of research is rooted in other norms and methodologies, it cannot be considered ‘lesser than’ or ‘unimportant’. Even more, I want to advocate for the idea that we can benefit from learning about other scientific disciplines and can be inspired by an idea which at first glance may appear completely unrelated to our own research.